
Last updated at Dec. 16, 2024 by Teachoo


Transcript
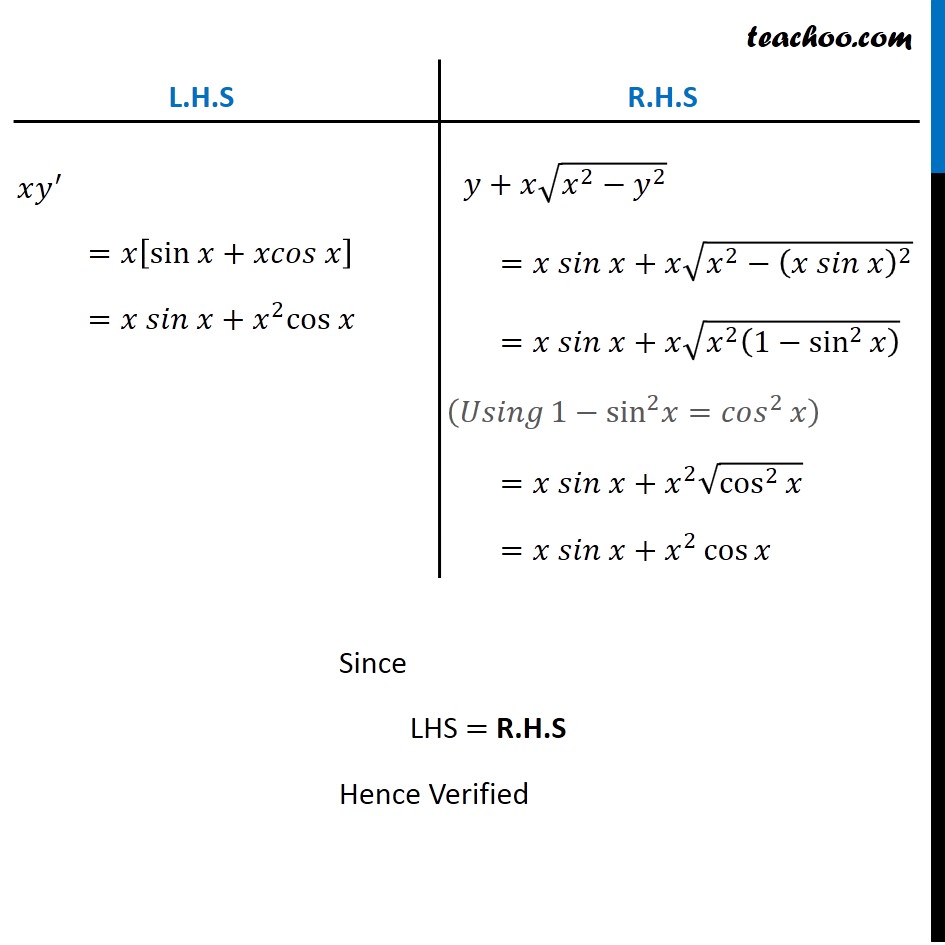
Ex 9.2, 6 Verify that the given functions (explicit or implicit) is a solution of the corresponding differential equation : 𝑦=𝑥 sin𝑥: 𝑥𝑦^′=𝑦+𝑥√(𝑥^2−𝑦^2 ) (𝑥≠0 𝑎𝑛𝑑 𝑥>𝑦 𝑜𝑟 𝑥<−𝑦 ) 𝑦=𝑥 sin𝑥 𝑑𝑦/𝑑𝑥=(𝑑(𝑥 sin𝑥))/𝑑𝑥 =𝑑(𝑥)/𝑑𝑥.sin𝑥+𝑥.𝑑(sin𝑥 )/𝑑𝑥 =1.sin𝑥+𝑥(cos𝑥 ) =sin𝑥+𝑥cos𝑥 Now, we have to verify 𝑥𝑦^′=𝑦+𝑥√(𝑥^2−𝑦^2 ) L.H.S 𝑥𝑦^′ =𝑥[sin 𝑥+𝑥𝑐𝑜𝑠 𝑥] =𝑥 𝑠𝑖𝑛 𝑥+𝑥^2 cos 𝑥 R.H.S 𝑦+𝑥√(𝑥^2−𝑦^2 ) =𝑥 𝑠𝑖𝑛 𝑥+𝑥√(𝑥^2−(𝑥 𝑠𝑖𝑛 𝑥)^2 ) =𝑥 𝑠𝑖𝑛 𝑥+𝑥√(𝑥^2 (1−sin^2𝑥 ) ) =𝑥 𝑠𝑖𝑛 𝑥+𝑥^2 √(cos^2𝑥 ) =𝑥 𝑠𝑖𝑛 𝑥+𝑥^2 cos𝑥 Since LHS = R.H.S Hence Verified