Ex 3.1, 6 - Chapter 3 Class 10 Pair of Linear Equations in Two Variables (Important Question)

Transcript

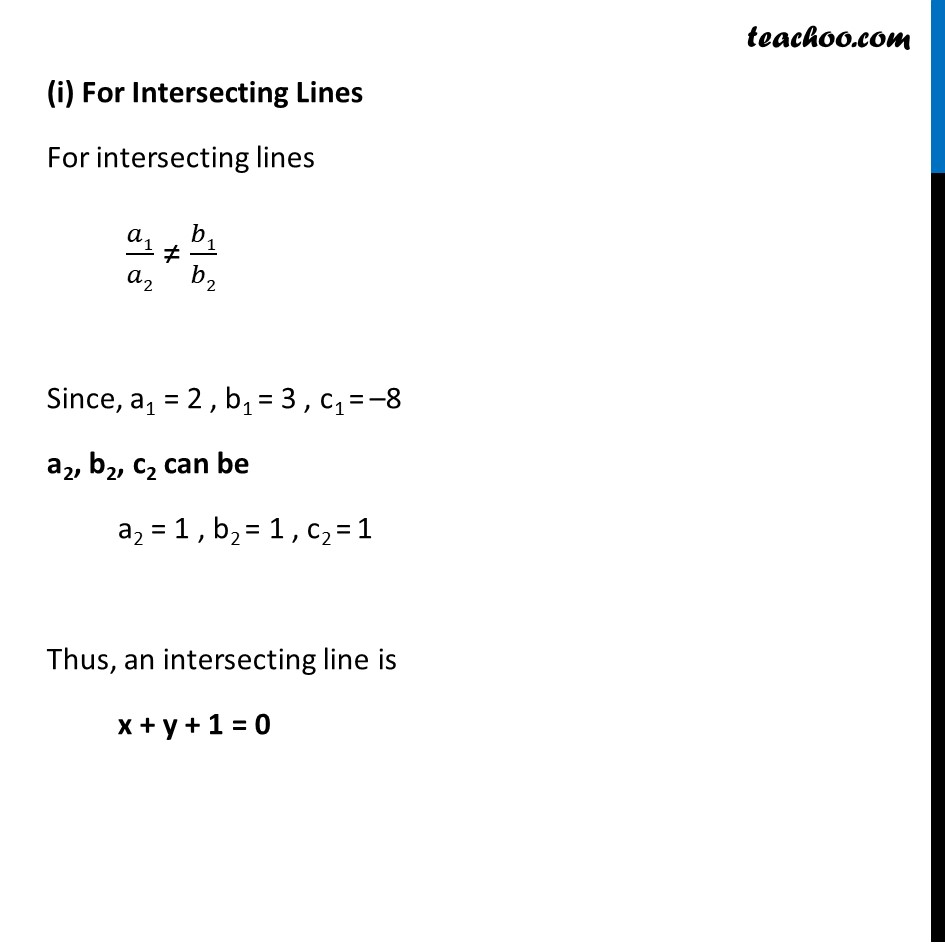
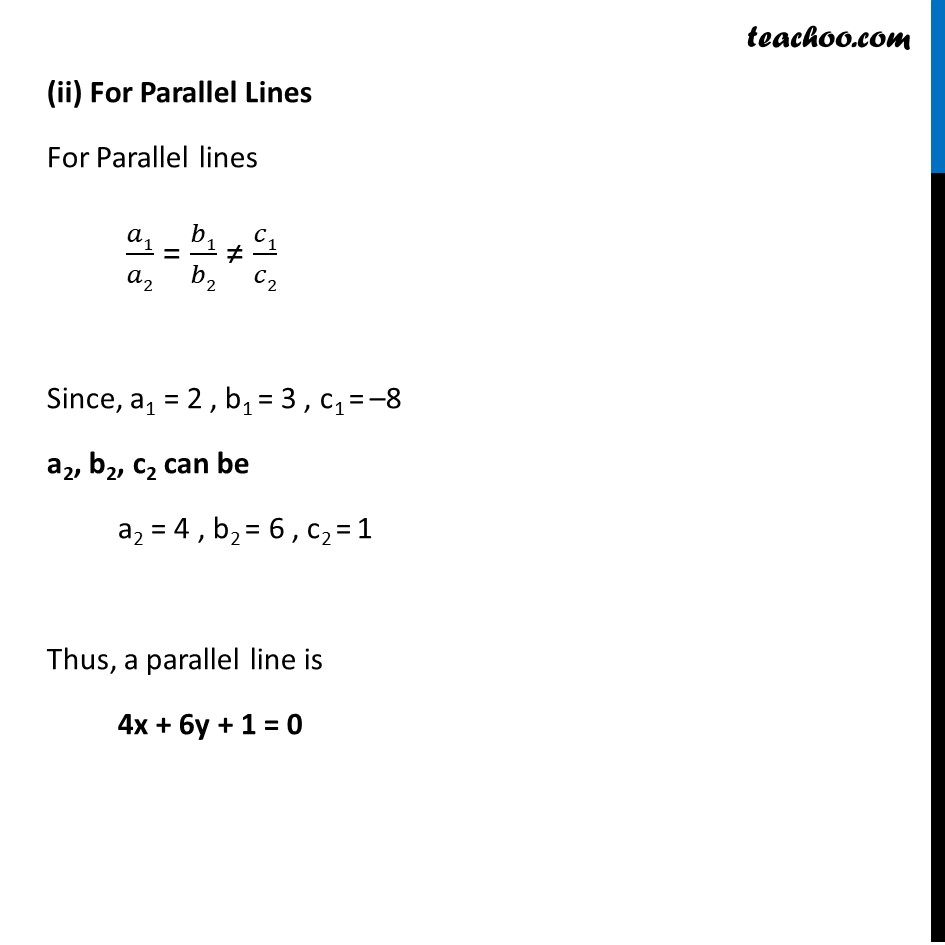
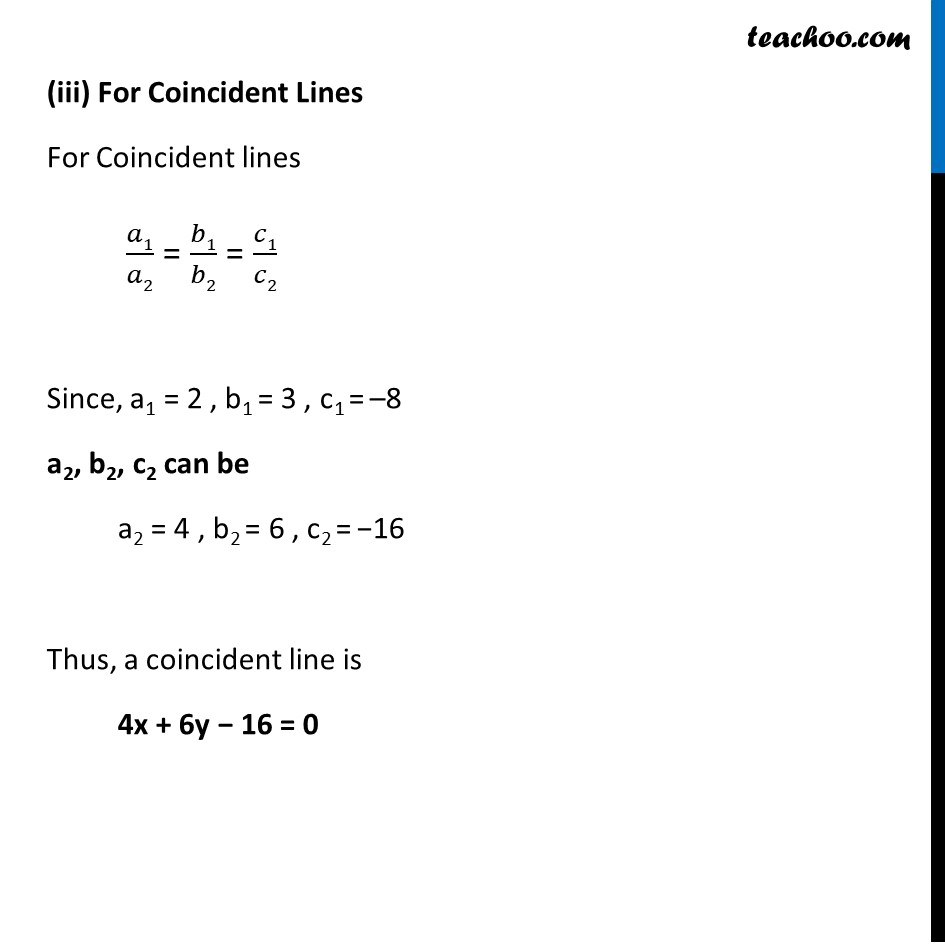
Ex 3.1, 6 Given the linear equation 2x + 3y – 8 = 0, write another linear equation in two variables such that the geometrical representation of the pair so formed is: (i) intersecting lines (ii) parallel lines (iii) coincident lines Given equation 2x + 3y − 8 = 0 Therefore, a1 = 2 , b1 = 3 , c1 = –8 (i) For Intersecting Lines For intersecting lines 𝑎1/𝑎2 ≠ 𝑏1/𝑏2 Since, a1 = 2 , b1 = 3 , c1 = –8 a2, b2, c2 can be a2 = 1 , b2 = 1 , c2 = 1 Thus, an intersecting line is x + y + 1 = 0 (ii) For Parallel Lines For Parallel lines 𝑎1/𝑎2 = 𝑏1/𝑏2 ≠ 𝑐1/𝑐2 Since, a1 = 2 , b1 = 3 , c1 = –8 a2, b2, c2 can be a2 = 4 , b2 = 6 , c2 = 1 Thus, a parallel line is 4x + 6y + 1 = 0 (iii) For Coincident Lines For Coincident lines 𝑎1/𝑎2 = 𝑏1/𝑏2 = 𝑐1/𝑐2 Since, a1 = 2 , b1 = 3 , c1 = –8 a2, b2, c2 can be a2 = 4 , b2 = 6 , c2 = −16 Thus, a coincident line is 4x + 6y − 16 = 0