Ex 4.3, 1 (iii) - Chapter 4 Class 10 Quadratic Equations

Transcript

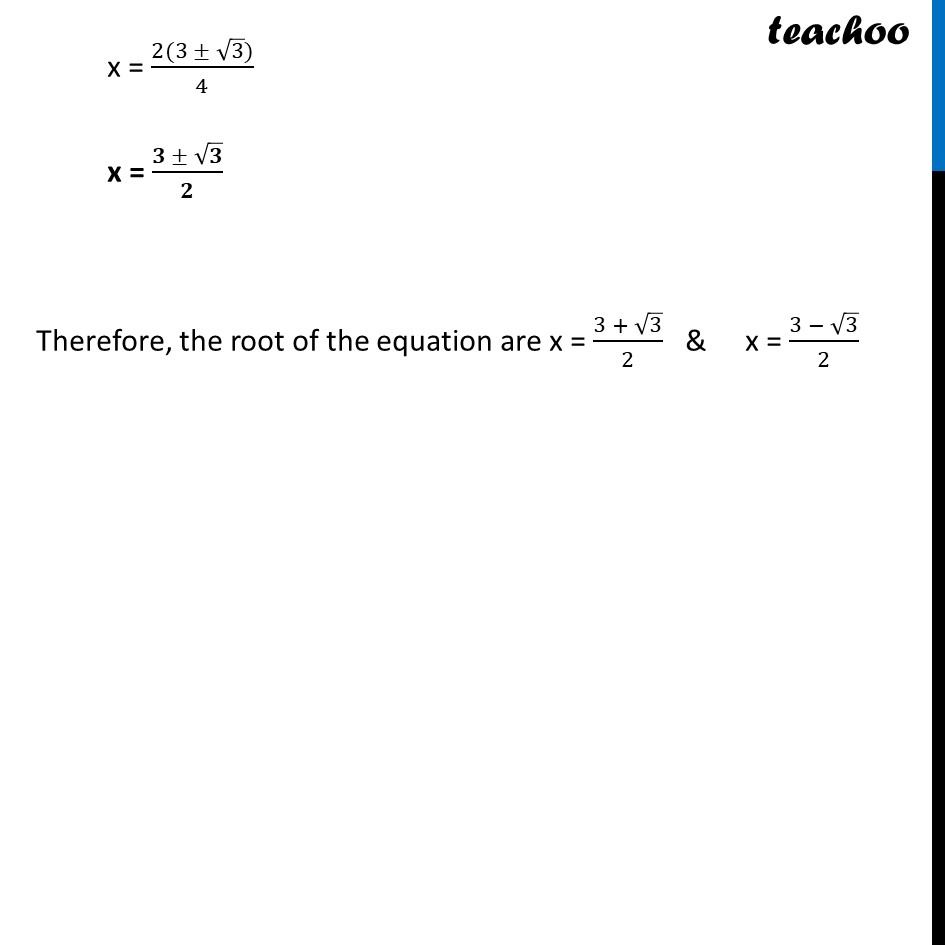
Ex 4.3, 1 Find the nature of the roots of the following quadratic equations. If the real roots exist, find them: (iii) 2x2 – 6x + 3 = 0 2x2 – 6x + 3 = 0 Comparing equation with ax2 + bx + c = 0 a = 2, b = –6 , c = 3 We know that D = b2 – 4ac = (–6)2 – 4 × 𝟐 × 𝟑 = (–6) × (–6) – 4 × 2 × 3 = 36 – 24 = 12 Since D > 0 There are 2 distinct real roots Now using quadratic formula to find roots x = (− 𝑏 ± √𝐷)/2𝑎 Putting values x = (−(− 𝟔) ± √𝟏𝟐)/(𝟐 × 𝟐) x = (+ 6 ± √12)/4 x = (6 ± √(4 × 3))/4 x = (6 ± √(4 )× √3)/4 x = (𝟔 ± 𝟐 ×√𝟑)/𝟒 x = (2(3 ± √3))/4 x = (𝟑 ± √𝟑)/𝟐 Therefore, the root of the equation are x = (3 + √3)/2 & x = (3 − √3)/2