Example 14 - Chapter 13 Class 12 Probability
Last updated at Dec. 16, 2024 by Teachoo
Independent events
Ex 13.2, 1
You are here
You are here
Chapter 13 Class 12 Probability
Concept wise
- Conditional Probability - Values given
- Conditional Probability - Statement
- Multiplication rule of probability
-
Independent events
- Basic Probability
- Theorem of total probability
- Bayes theorem
- Random Variable - Defination
- Probability distribution
- Mean or Expectation of random variable
- Variance and Standard Deviation of a Random Variable
- Bernoulli Trials
- Binomial Distribution
Transcript
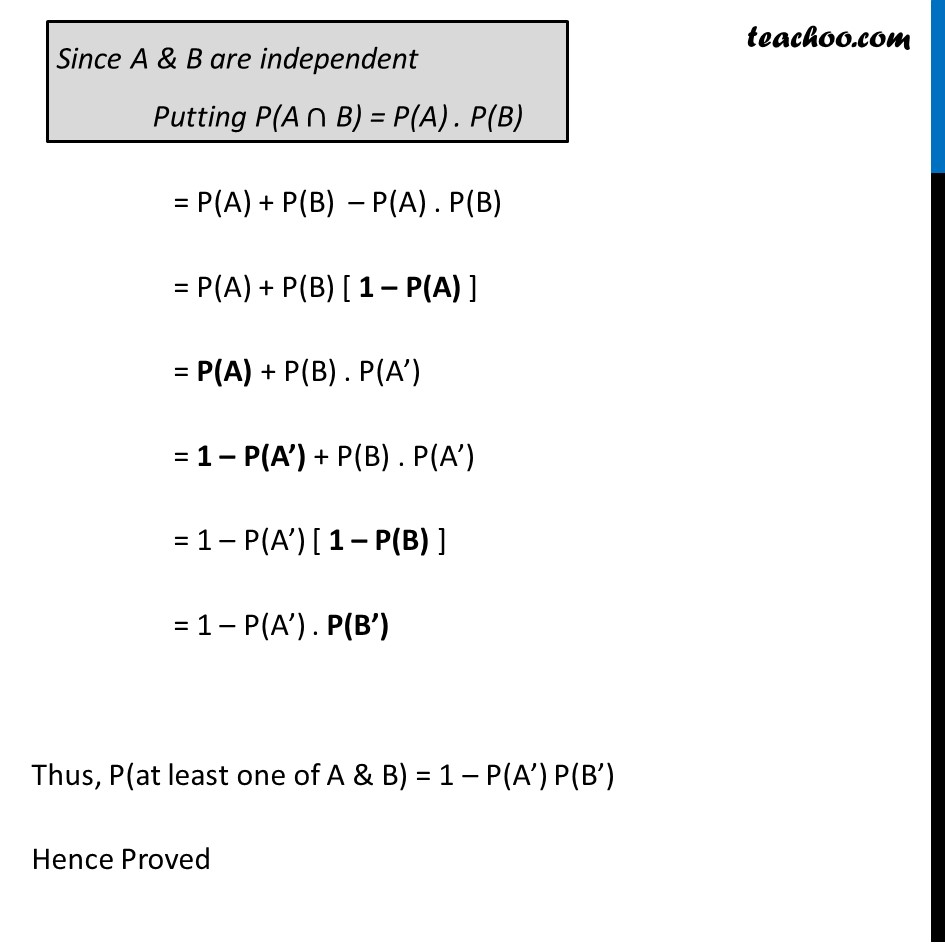
Example 14 If A and B are two independent events, then the probability of occurrence of at least one of A and B is given by 1– P(A′) P(B′) Since events A and B are independent ∴ P(A ∩ B) = P(A) . P(B) Now, Probability of occurrence of at least one of A and B = Probability of occurrence of only A + Probability of occurrence of only B + Probability of occurrence of A and B = Probability of occurrence of A or B = P(A ∪ B) = P(A) + P(B) – P(A ∩ B) Since A & B are independent Putting P(A ∩ B) = P(A) . P(B) = P(A) + P(B) – P(A) . P(B) = P(A) + P(B) [ 1 – P(A) ] = P(A) + P(B) . P(A’) = 1 – P(A’) + P(B) . P(A’) = 1 – P(A’) [ 1 – P(B) ] = 1 – P(A’) . P(B’) Thus, P(at least one of A & B) = 1 – P(A’) P(B’) Hence Proved
