Question 11 - Coplanarity of 2 lines - Chapter 11 Class 12 Three Dimensional Geometry

Transcript

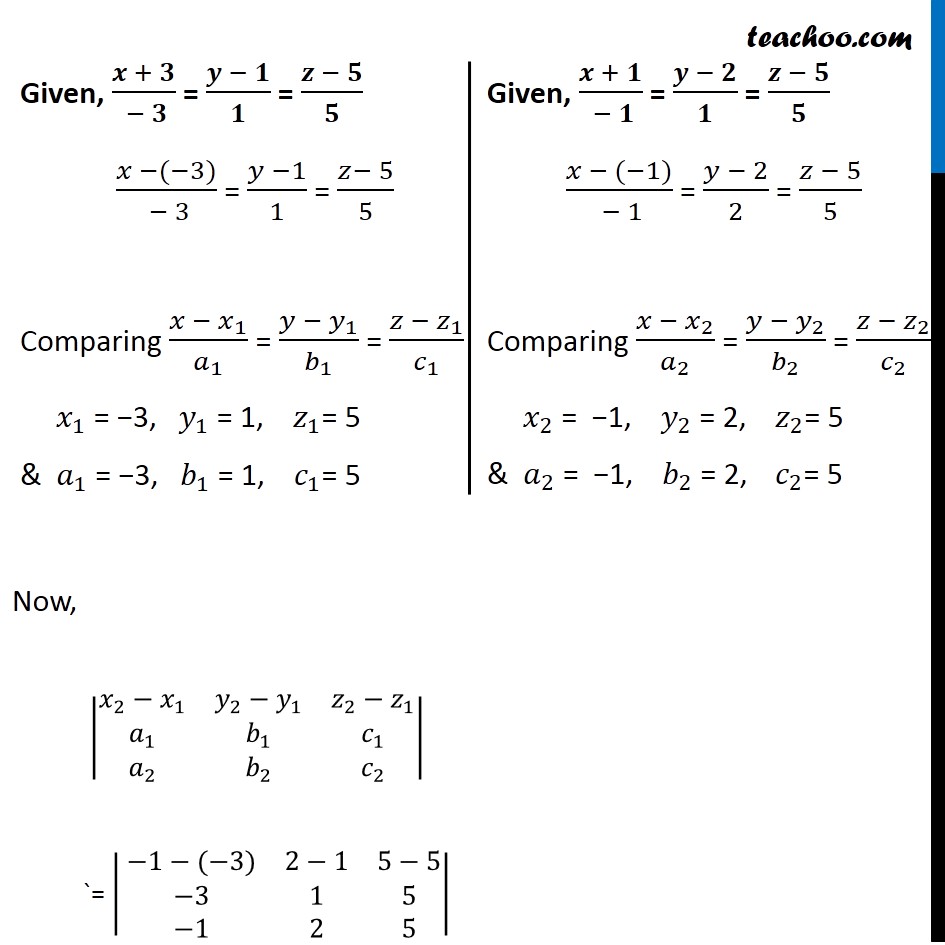
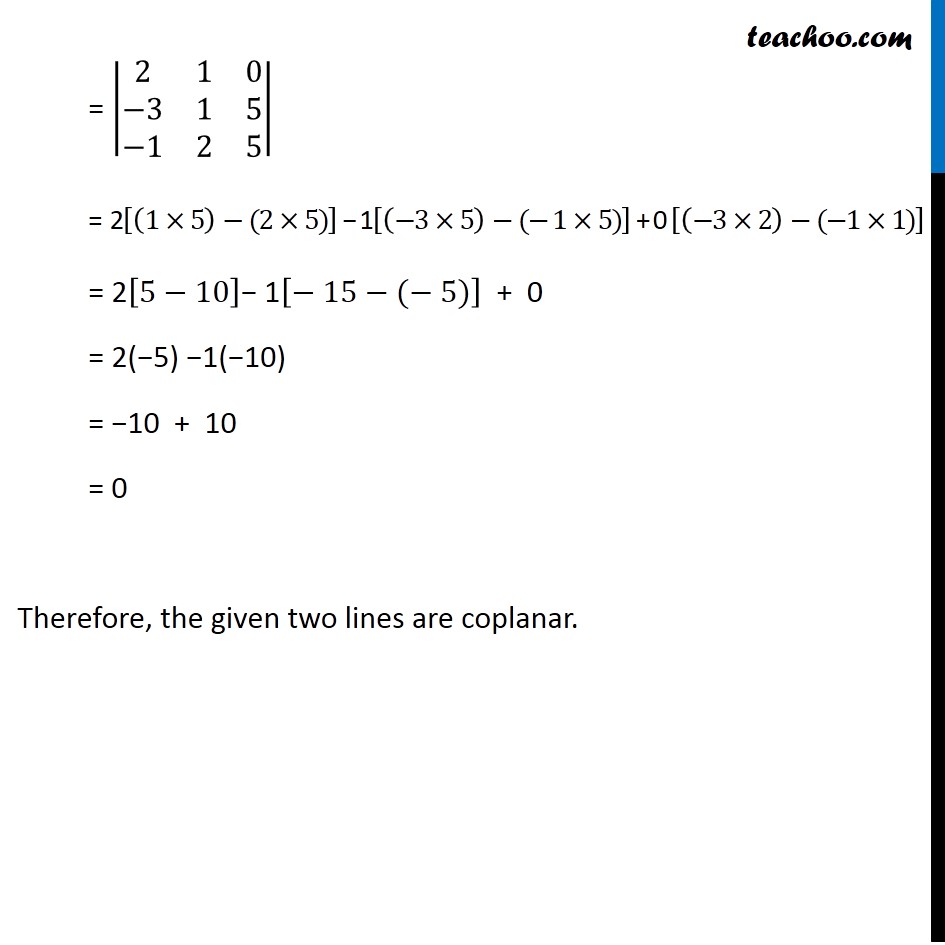
Question 11 Show that the lines (𝑥 + 3)/( −3) = (𝑦 − 1)/1 = (𝑧 − 5)/5 and (𝑥 + 1)/( −1) = (𝑦 − 2)/2 = (𝑧 − 5)/5 are coplanar. Two lines (𝑥 − 𝑥_1)/𝑎_1 = (𝑦 − 𝑦_1)/𝑏_1 = (𝑧 − 𝑧_1)/𝑐_1 and (𝑥 − 𝑥_2)/𝑎_2 = (𝑦 − 𝑦_2)/𝑏_2 = (𝑧 − 𝑧_2)/𝑐_2 are coplanar if |■8(𝒙_𝟐−𝒙_𝟏&𝒚_𝟐−𝒚_𝟏&𝒛_𝟐−𝒛_𝟏@𝒂_𝟏&𝒃_𝟏&𝒄_𝟏@𝒂_𝟐&𝒃_𝟐&𝒄_𝟐 )| = 0 Given, the two lines are Given, (𝒙 + 𝟑)/( − 𝟑) = (𝒚 − 𝟏)/𝟏 = (𝒛 − 𝟓)/𝟓 (𝑥 −(−3))/( − 3) = (𝑦 −1)/1 = (𝑧− 5)/5 Comparing (𝑥 − 𝑥_1)/𝑎_1 = (𝑦 − 𝑦_1)/𝑏_1 = (𝑧 − 𝑧_1)/𝑐_1 𝑥_1 = −3, 𝑦_1 = 1, 𝑧_1= 5 & 𝑎_1 = −3, 𝑏_1 = 1, 𝑐_1= 5 Given, (𝒙 + 𝟏)/( − 𝟏) = (𝒚 − 𝟐)/𝟏 = (𝒛 − 𝟓)/𝟓 (𝑥 − (−1))/( − 1) = (𝑦 − 2)/2 = (𝑧 − 5)/5 Comparing (𝑥 − 𝑥_2)/𝑎_2 = (𝑦 − 𝑦_2)/𝑏_2 = (𝑧 − 𝑧_2)/𝑐_2 𝑥_2 = −1, 𝑦_2 = 2, 𝑧_2= 5 & 𝑎_2 = −1, 𝑏_2 = 2, 𝑐_2= 5 Now, |■8(𝑥_2−𝑥_1&𝑦_2−𝑦_1&𝑧_2−𝑧_1@𝑎_1&𝑏_1&𝑐_1@𝑎_2&𝑏_2&𝑐_2 )| `= |■8( −1−(−3)&2−1&5−5@ −3&1&5@ −1&2&5)| = |■8(2&1&0@−3&1&5@−1&2&5)| = 2[(1×5)−(2×5)] − 1[(−3×5)−(− 1×5)] + 0 [(−3×2)−(−1×1)] = 2[5−10]− 1[− 15−(− 5)] + 0 = 2(−5) −1(−10) = −10 + 10 = 0 Therefore, the given two lines are coplanar.