Example 3 - Chapter 1 Class 12 Relation and Functions
Last updated at Dec. 16, 2024 by Teachoo
To prove relation reflexive, transitive, symmetric and equivalent
What is reflexive, symmetric, transitive relation?
You are here
You are here
Chapter 1 Class 12 Relation and Functions
Concept wise
- Relations - Definition
- Empty and Universal Relation
-
To prove relation reflexive, transitive, symmetric and equivalent
- Finding number of relations
- Function - Definition
- To prove one-one & onto (injective, surjective, bijective)
- Composite functions
- Composite functions and one-one onto
- Finding Inverse
- Inverse of function: Proof questions
- Binary Operations - Definition
- Whether binary commutative/associative or not
- Binary operations: Identity element
- Binary operations: Inverse
Transcript
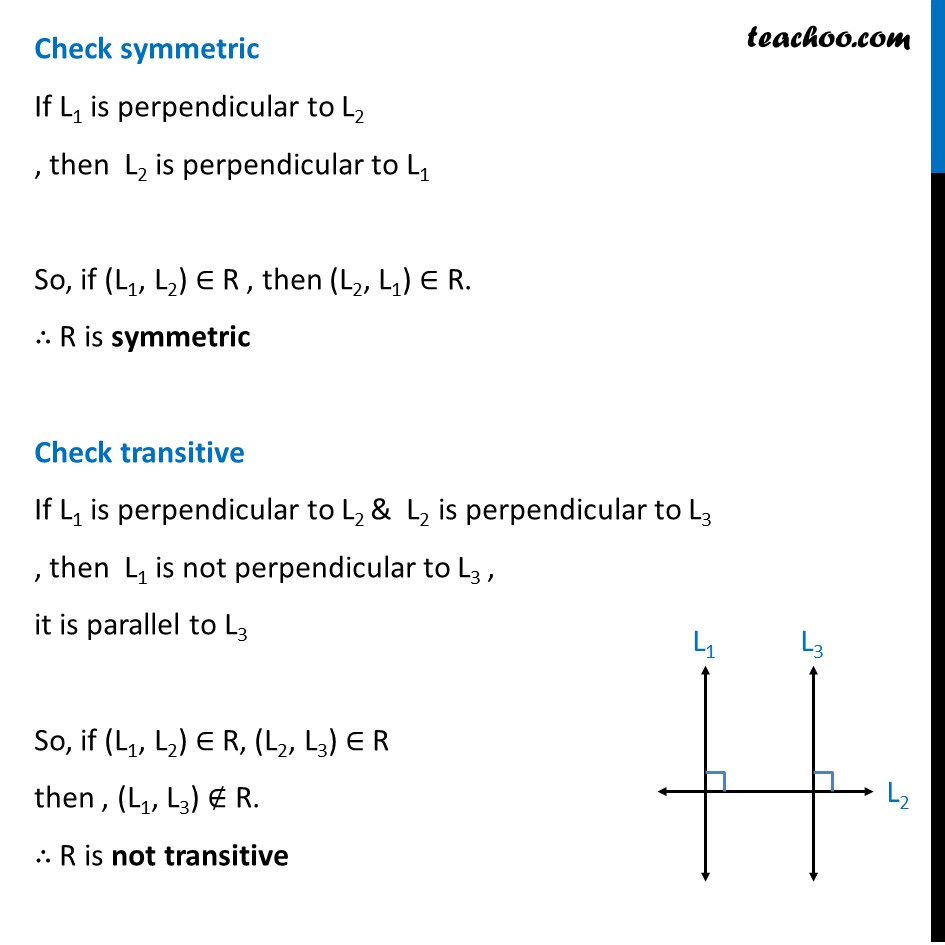
Example 3 Let L be the set of all lines in a plane and R be the relation in L defined as R = {(L1, L2) : L1 is perpendicular to L2}. Show that R is symmetric but neither reflexive nor transitive. R = {(L1, L2) : L1 is perpendicular to L2} Check reflexive If R is reflexive, then (L, L) ∈ R Line L cannot be perpendicular to itself So, line L is not perpendicular to line L So, (L, L) ∉ R. ∴ R is not reflexive Check symmetric If L1 is perpendicular to L2 , then L2 is perpendicular to L1 So, if (L1, L2) ∈ R , then (L2, L1) ∈ R. ∴ R is symmetric Check transitive If L1 is perpendicular to L2 & L2 is perpendicular to L3 , then L1 is not perpendicular to L3 , it is parallel to L3 So, if (L1, L2) ∈ R, (L2, L3) ∈ R then , (L1, L3) ∉ R. ∴ R is not transitive
